
Similarity in the 3rd Degree
By: John Vereen
Prove
the pedal triangle of the pedal triangle of the pedal triangle of a point is
similar to the original triangle.
In
order to prove that a 3rd degree pedal triangle is similar to the
original triangle, we must first describe what it means for two triangles to be
similar. Two triangles are similar if:
1)
The triangles
have the same angle measures for each of the three respective angles.
2)
The triangles
have proportional side lengths.
In order to prove that the
third order pedal triangle is similar to the original triangle, we must prove
one of these two facts about similar triangles.
So, Let’s prove that third order
pedal triangles have the same angle measures as the original triangle.
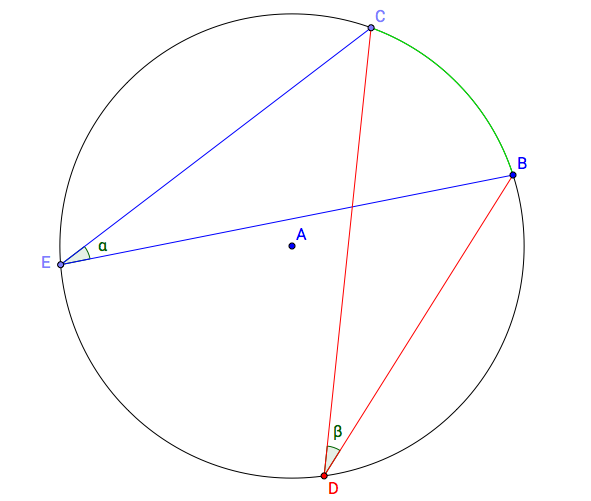
There is a theorem (depicted above) we
discussed in my MATH 5200 class that will be crucial in
proving this fact. This theorem is known as the “Angles in a Circle theorem” or
“Angles subtended on the same Arc theorem.” Basically, this theorem states that angles formed from two points in the same arc on the
circumference of a circle are equal to other angles formed from those two
points.
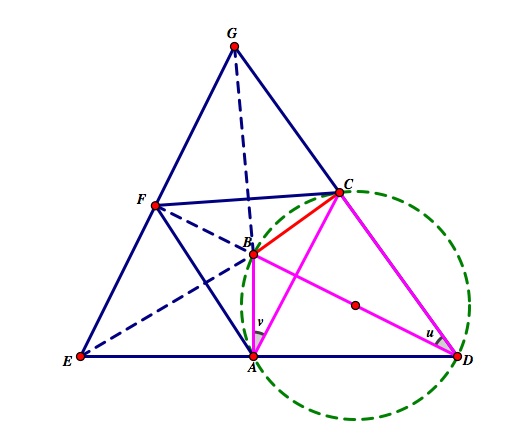
Above,
we have a triangle EDG and a first order pedal triangle ACF depicted. We can
form a circle that contains points A,B,C, and D along
the circumference. Using the “Angles in a Circle” Theorem described above, we
can see that points A and D are in the same arc length BC. Thus, we can say
that angle BAC = BDC (u = v).
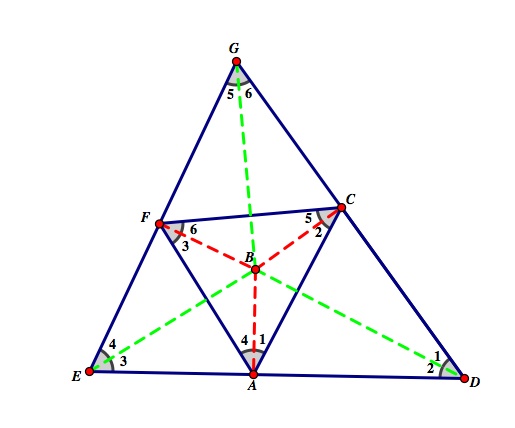
Similarly,
this theorem can be applied to six different pairs of congruent angles formed
by the original triangle, the pedal triangle, and the points from the triangle
vertices to the pedal point. The angles with the same numerical label are
congruent to one another by the “Angles in a Circle” theorem.

Once
we apply this theorem to the second and third order, we now look at the angles
that form the original triangle and the third order pedal triangle (the
smallest blue triangle and the largest blue triangle). We see that, in the
original triangle, the first angle D is
composed of angles 1 and 2, the second angle at E is composed of angles 3 and 4, and the third angle at G is composed of angles 5 and 6. The
three angles that compose the third order pedal triangle are also the pairs 1
and 2, 3 and 4, 5 and 6. Thus, we have proven, using the “Angles in a Circle”
theorem, that the pedal triangle of
the pedal triangle of the pedal triangle of a point is similar to the original
triangle.